


1. Four 5 ohm resistors are connected in a series. The potential difference across the circuit is 40 V. (A.) What is the equivalent resistance of the circuit? (B.) What is the current in each resistor?
a) Req = R1 + R2 + R3 + R4
Req = 5 + 5 + 5 + 5
Req =20 ohms
b) Ieq = V / Req
Ieq = 40 / 20
Ieq = 2 amps running through each since they are in series
2. If the resistors above are connected in parallel instead, (A.) What is the equivalent resistance of the circuit? (B.) What is the current in each resistor?
a) 1/Req = 1/R1 + 1/R2 + 1/R3 + 1/R4
1/Req = 1/5 + 1/5 + 1/5 + 1/5
1/Req = 0.8
Req = 1.25 ohms
b) Ieq = V / Req
Ieq = 40 / 1.25
Ieq = 32 amps
I1 = V / R1
I1 = 40 / 5
I1 = 8 amps
I1 = I2 = I3 = I4 = 8 amps
Because all the resistors are equal and the voltage is the
same for each.
3.

In the circuit above, find the values for (A.) I1, (B.) I2, and (C.) I3.
I1 = I2 + I3 loop 1 12 - 4*I1 - 2*I3 = 0 loop 2 2*I3 - 3*I2 = 0 Matrix A Matrix B 1 -1 -1 0 -4 0 -2 -12 0 -3 2 0 A-1 * B = C Matrix C I1 = 2.31 amps I2 = 0.92 amps I3 = 1.38 amps
4.
An uncharged capacitor and a resitor are connected in series to a battery as depicted above. If E = 20 V, C = 10 x 10-6, and R = 5 x 106, (A.) find the time constant of the circuit, (B.) the maximum charge on the capacitor, (C.) the maximum current in the circuit, and (D.) charge and current as a function of time.
a) T = R * C T = (5 x 106) * (10 x 10-6) T = 50 s b) Qmax = E * C Qmax = 20 * (10 x 10-6) Qmax = 2 x 10-4 C c) Imax = E / R Imax = 20 / (5 x106) Imax = 4 x 10-6 amps d) Q(t) = Qmax * (1 - e(-t/T)) Q(t) = (2 x 10-4) * (1 - e(-t/50)) Q(t) = (2 x 10-4) -(2 x10-4e(-t/50))
5.
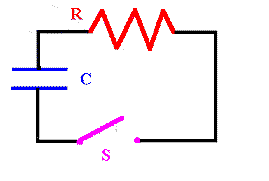
Consider a capacitor C being discharged through a resistor R as depicted above. (A.) After how many time constants will the charge on the capacitor drop to one-third of its initial value? (B.) The energy stored in the capacitor decreases with time as it discharges. After how many time constants will this stored energy reduce to one-third of its initial value?
a) Q = Qmax*(e(-t/T)) 1/3 = 1*(e(-t/T)) b) U = .5*Q*V = .5*C*V2
6. Consider a proton near the magnetic equator. In which direction will it tend to be deflected if its velocity is directed (A.) downward, (B.) westward, (C.) northward, or (D.) southeastward?
a)westward b)zero deflection c)upward d)downward
7. The magnetic field over a certain region is given by B = (5i-13j) T. An electron moves in the field with a velocity v = (-4i+5j-9k) m/s. Write out, in unit-vector notation, the force exerted on the electron by the magnetic field.
F = q*V x B F = (-1.602 x 10-19)[(-4i+5j-9k) x (5i-13j)] F =
8. A wire carries a steady current of 3.4 A. A straight section of the wire, with a length of 0.65 m along the x, lies within a uniform magnetic field, B = (2.6k) T. If the current flows in the +x direction, what is the magnetic force on the section of wire?
F = I*L x B F = 3.4*.65j x 2.6k F = (-5.75j) newtons
9. A conducting rod of length l moves on two horizontal frictionless rails. If a constant force of 2 N moves the bar at 3 m/s through a magnetic field B which is into the paper, (A.) what is the current through an 9 ohm resistor? (B.) What is the rate of energy dissipation in the resistor? (C.) What is the mechanical power delivered by the force F?
a) E = B*l*v F = B*I*l I*R = B*l*v B = F/(I*l) I*R = [F/(I*l)]*l*v I2 = [F*v] / R I2 = [2*3] / 9 I2 = 0.6667 I = 0.82 amps b) Pe = I2*R Pe = (0.82)2*9 Pe = 6.05 watts c) Pmech = Pe, because of energy conservation Pmech = 6.05 watts
10. A square coil (40 cm X 40 cm) that consists of 200 turns of wire rotates about a vertical axis 2250 rpm. The horizontal component of the earth's magnetic field at the location of the loop is 4 x 10-4 T. Calculate the maximum emf induced in the coil by the earth's field.
w = (2250 rev/min) * (2pi rad/1 rev) * (1 min/60 s) w = 236 rad/s A = .4 * .4 A = .16 m2 Emax = n*A*B*w Emax = 200*.16*(4 x 10-4)*236 Emax = 3.02 volts
